剑指 Offer II 110. 所有路径｜797. 所有可能的路径：

给定一个有 n 个节点的有向无环图，用二维数组 graph 表示，请找到所有从 0 到 n-1 的路径并输出（不要求按顺序）。

graph 的第 i 个数组中的单元都表示有向图中 i 号节点所能到达的下一些结点（译者注：有向图是有方向的，即规定了 a→b 你就不能从 b→a ），若为空，就是没有下一个节点了。

样例 1：

输入：
	graph = [[1,2],[3],[3],[]]
	
输出：
	[[0,1,3],[0,2,3]]
	
解释：
	有两条路径 0 -> 1 -> 3 和 0 -> 2 -> 3

样例 2：

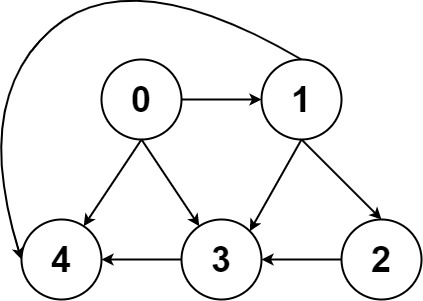
输入：
	graph = [[4,3,1],[3,2,4],[3],[4],[]]
	
输出：
	[[0,4],[0,3,4],[0,1,3,4],[0,1,2,3,4],[0,1,4]]

样例 3：

输入：
	graph = [[1],[]]
	
输出：
	[[0,1]]

样例 4：

输入：
	graph = [[1,2,3],[2],[3],[]]
	
输出：
	[[0,1,2,3],[0,2,3],[0,3]]

样例 5：

输入：
	graph = [[1,3],[2],[3],[]]
	
输出：
	[[0,1,2,3],[0,3]]

提示：

• n == graph.length
• 2 <= n <= 15
• 0 <= graph[i][j] < n
• graph[i][j] != i
• 保证输入为有向无环图 (GAD)

分析

• 这道算法题幸好是 无环图 ，要不然就没那么简单了。
• 遍历路径，找到所有可以到达终点节点的路径就是结果。
• 大部分语言的题解都是用的动态数据结构，所以可以规避一个问题，那就是你要提前知道结果集的最大数量。
• C语言由于是手动去申请内存，所以需要知道结果集的最大数量，当然去申请很大的内存肯定就够放，但是本着算法精神，我们应该申请刚好够用的内存。
• 提示中说 保证输入为有向无环图 (GAD) ，所以我们可以认为节点间一定有着某种排列的顺序，从头到尾怎样可以有最多的路径呢，那就是在保证没有环路的情况下，所有节点都尽可能多的连接着其他节点。
• 开始节点可以直接到终点节点…途径任何一个节点都能到终点…途径任何二个节点都能到终点…以此类推，所以中间的节点就成了可以任意组合，一共多少种组合，每个节点都是经过或者不经过两种可能，由于头尾的节点是必经经过的，所以最多结果集的数量就是 (n - 2)² , 也就是 1 << n - 2。

题解

java

class Solution {
    public List<List<Integer>> allPathsSourceTarget(int[][] graph) {
        List<List<Integer>> ans   = new ArrayList<>();
        Deque<Integer>      stack = new ArrayDeque<>();
        stack.offerLast(0);
        dfs(graph, stack, ans);
        return ans;
    }

    private void dfs(int[][] graph, Deque<Integer> stack, List<List<Integer>> ans) {
        if (stack.peekLast() == graph.length - 1) {
            ans.add(new ArrayList<>(stack));
            return;
        }
        for (int to : graph[stack.peekLast()]) {
            stack.offerLast(to);
            dfs(graph, stack, ans);
            stack.pollLast();
        }
    }
}

c

void dfs(int **graph, int *graphColSize, int *returnSize, int **returnColumnSizes, int n, int *stack, int stackSize, int **ans) {
    int last = stack[stackSize - 1];
    if (last == n) {
        int *row = malloc(sizeof(int) * stackSize);
        memcpy(row, stack, sizeof(int) * stackSize);
        ans[*returnSize] = row;
        (*returnColumnSizes)[(*returnSize)++] = stackSize;
        return;
    }
    for (int i = 0; i < graphColSize[last]; ++i) {
        int to = graph[last][i];
        stack[stackSize] = to;
        dfs(graph, graphColSize, returnSize, returnColumnSizes, n, stack, stackSize + 1, ans);
    }
}

/**
 * Return an array of arrays of size *returnSize.
 * The sizes of the arrays are returned as *returnColumnSizes array.
 * Note: Both returned array and *columnSizes array must be malloced, assume caller calls free().
 */
int** allPathsSourceTarget(int** graph, int graphSize, int* graphColSize, int* returnSize, int** returnColumnSizes){
    *returnSize = 0;
    *returnColumnSizes = malloc(sizeof(int) * (1 << graphSize - 2));
    int **ans = malloc(sizeof(int *) * (1 << graphSize - 2));
    int *stack = malloc(sizeof(int) * graphSize);
    stack[0] = 0;
    dfs(graph, graphColSize, returnSize, returnColumnSizes, graphSize - 1, stack, 1, ans);
    return ans;
}

c++

class Solution {
private:
    void dfs(vector<vector<int>>& graph, vector<int>& stack, vector<vector<int>>& ans) {
        if (stack.back() == graph.size() - 1) {
            ans.push_back(stack);
            return;
        }
        for (auto& to : graph[stack.back()]) {
            stack.push_back(to);
            dfs(graph, stack, ans);
            stack.pop_back();
        }
    }
public:
    vector<vector<int>> allPathsSourceTarget(vector<vector<int>>& graph) {
        vector<vector<int>> ans;
        vector<int> stack;
        stack.push_back(0);
        dfs(graph, stack, ans);
        return ans;
    }
};

python

class Solution:
    def allPathsSourceTarget(self, graph: List[List[int]]) -> List[List[int]]:
        ans = list()
        stack = list()

        def dfs():
            last = stack[len(stack) - 1]
            if last == len(graph) - 1:
                ans.append(stack[:])
                return

            for to in graph[last]:
                stack.append(to)
                dfs()
                stack.pop()

        stack.append(0)
        dfs()
        return ans
        

go

func allPathsSourceTarget(graph [][]int) (ans [][]int) {
	n := len(graph) - 1
	stack := []int{0}
	var dfs func()
	dfs = func() {
		last := stack[len(stack)-1]
		if last == n {
			ans = append(ans, append([]int{}, stack...))
			return
		}
		for _, to := range graph[last] {
			stack = append(stack, to)
			dfs()
			stack = stack[:len(stack)-1]
		}
	}
	dfs()
	return
}

rust

impl Solution {
    pub fn all_paths_source_target(graph: Vec<Vec<i32>>) -> Vec<Vec<i32>> {
        let mut ans: Vec<Vec<i32>> = Vec::new();
        let mut stack: Vec<i32> = Vec::new();
        stack.push(0);
        Solution::dfs(&graph, graph.len() as i32 - 1, &mut stack, &mut ans);
        ans
    }

    fn dfs(graph: &Vec<Vec<i32>>, n: i32, stack: &mut Vec<i32>, ans: &mut Vec<Vec<i32>>) {
        let last = stack[stack.len() - 1];
        if last == n {
            ans.push(stack.clone());
            return;
        }
        graph[last as usize].iter().for_each(|to| {
            stack.push(to.clone());
            Solution::dfs(graph, n, stack, ans);
            stack.pop();
        });
    }
}

原题传送门：https://leetcode-cn.com/problems/bP4bmD/

原题传送门：https://leetcode-cn.com/problems/all-paths-from-source-to-target/

非常感谢你阅读本文~
放弃不难，但坚持一定很酷~
希望我们大家都能每天进步一点点~
本文由 二当家的白帽子：https://bbs.huaweicloud.com/community/usersnew/id_1628396583336561 博客原创~