前言

Hello！小伙伴！
非常感谢您阅读海轰的文章，倘若文中有错误的地方，欢迎您指出～
 
自我介绍 ଘ(੭ˊᵕˋ)੭
昵称：海轰
标签：程序猿｜C++选手｜学生
简介：因C语言结识编程，随后转入计算机专业，有幸拿过一些国奖、省奖…已保研。目前正在学习C++/Linux/Python
学习经验：扎实基础 + 多做笔记 + 多敲代码 + 多思考 + 学好英语！
 
机器学习小白阶段
文章仅作为自己的学习笔记 用于知识体系建立以及复习
知其然 知其所以然！

往期文章

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（1）：集合与映射

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（2）：线性空间定义及其性质

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（3）：线性空间的基与坐标

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（4）：基变换与坐标变换

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（5）：线性子空间

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（6）：子空间的交与和

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（7）：欧氏空间

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（8）：标准正交基与Gram-Schmidt过程

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（9）：正交补与投影定理

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（10）：线性变换定义

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（11）：线性变换的矩阵表示

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（12）：相似形理论

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（13）：Hamliton-Cayley定理、最小多项式

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（14）：向量范数及其性质

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（15）：矩阵的范数

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（16）：向量和矩阵的极限

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（17）：函数矩阵的微分和积分

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（18）：方阵的幂级数

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（19）：不定积分（补充知识）

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（20）：方阵函数

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（21）：常用方阵函数的一些性质

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（22）：方阵函数在微分方程组中的应用

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（23）：常数项级数的概念和性质（补充知识）

【机器学习｜数学基础】Mathematics for Machine Learning系列之矩阵理论（24）：常数项级数的审敛法（补充知识））

幂级数

一、函数项级数的概念

定义：（函数项）无穷级数

如果给定一个定义在区间$I$上的函数列

$$u_1(x),u_2(x),u_3(x),...,u_n(x),...,$$

那么由这函数列所构成的表达式

$$u_1(x)+u_2(x)+u_3(x)+...+u_n(x)+...$$

称为定义在区间$I$上的（函数项）无穷级数，简称（函数项）级数

对于每一个确定的值$x_0\in I$，函数项级数成为常数项级数

$$u_1(x_0)+u_2(x_0)+u_3(x_0)+...+u_n(x_0)+...$$

这个级数可能收敛，也可能发散

如果这个级数收敛，就称点$x_0$是函数项级数的收敛点

如果这个级数发散，就称点$x_0$是函数项级数的发散点

函数项级数的收敛点的全体称为它的收敛域，发散点的全体称为它的发散域

幂级数及其收敛性

幂级数

各项都是常数乘以幂函数的函数项级数，即为幂级数，形式为

$$\sum _{n=0}^{\infty }{a}_n{x}^n=a_0+a_{1}x+a_2{x}^2+...+a_n{x}^n+....$$

其中常数$a_0,a_1,a_2,...,a_n,...$叫做幂级数的系数

定理1（阿贝尔定理）

推论

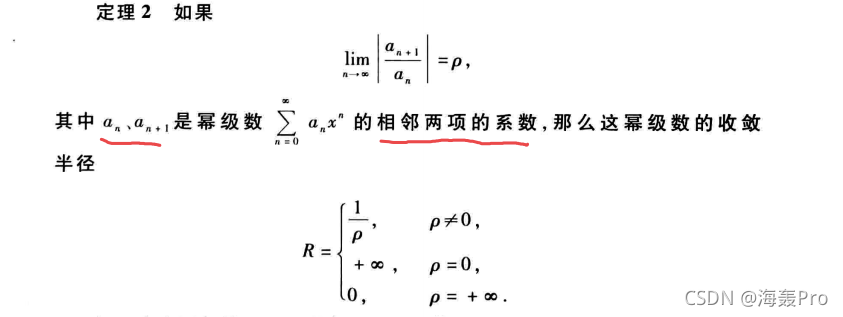
定理2

结语

说明：

• 参考于 课本《高等数学》
• 配合书中概念讲解 结合了自己的一些理解及思考

文章仅作为学习笔记，记录从0到1的一个过程

希望对您有一点点帮助，如有错误欢迎小伙伴指正

文章来源: haihong.blog.csdn.net，作者：海轰Pro，版权归原作者所有，如需转载，请联系作者。

原文链接：haihong.blog.csdn.net/article/details/121323497